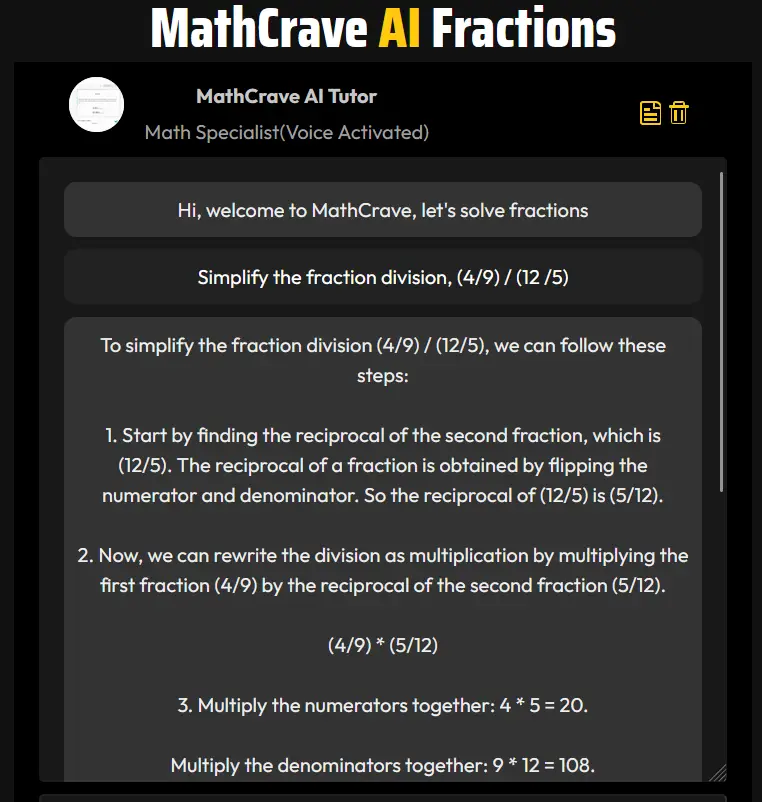
Mastering the techniques to add, subtract, multiply, and divide fractions, one can confidently solve various mathematical problems and build a solid mathematical foundation. MathCrave AI Fraction Solver does it.
Having a strong foundation in mathematics requires understanding the terminology of fractions. The terms numerator and denominator play crucial roles in comprehending the nature of fractions.
The top part of a fraction, known as the numerator, represents the quantity or value being considered. On the other hand, the bottom part of a fraction, known as the denominator, indicates the total number of equal parts into which a whole is divided.
3/9 where 3 is the numerator and 9, the denominator
9/8 where 9 is the numerator and 8, the denominator (improper fraction) since the 9 is greater than 8
3 (4/5) where 3 is the whole number 4, numerator and 5, the denominator ( mixed numbers)
It is important to differentiate between proper fractions and improper fractions. Proper fractions are those where the numerator is smaller than the denominator and always represent values less than one.
For example, 3/4 and 1/2 are proper fractions. Conversely, improper fractions have a numerator that is equal to or greater than the denominator and represent values equal to or greater than one. For instance, 5/4 and 7/3 are improper fractions. Another type of fraction is a mixed number, which combines a whole number and a proper fraction.
It is written in the form of a whole number, a space, and then the proper fraction. For example, 2 1/3 and 4 2/5 are mixed numbers. Adding and subtracting fractions is an essential skill in mathematics.
To add or subtract fractions, you need to ensure that the denominators are the same. If they are not, you must convert the fractions to equivalent fractions with a common denominator before performing the operation.
Multiplying two fractions involves multiplying the numerators together and then multiplying the denominators together. The resulting product is a simplified fraction. For instance, when multiplying 2/3 by 5/7, the numerator becomes 2 * 5 = 10 and the denominator becomes 3 * 7 = 21, resulting in the product 10/21.
To solve this Bodmas, enter the expression as shown below
[ 1(3/5) ] x [ 2(1/3) ] x [ 3(3/7) ]
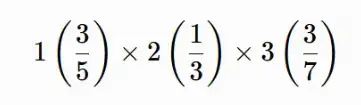
To solve this BODMAS, enter the expression as shown below
1/3 of ( 5(1/2) - 3(3/4) ) + ( 3(1/5) ÷ 4/5 - 1/2 )
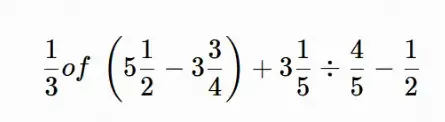
Dividing two fractions requires multiplying the first fraction by the reciprocal of the second fraction. The reciprocal of a fraction is obtained by swapping the numerator and denominator. For example, when dividing 2/3 by 5/7, you multiply 2/3 by 7/5. The numerator becomes 2 7 = 14 and the denominator becomes 3 5 = 15, resulting in the quotient 14/15.
9/2
28/12
Use sample expression to solve fraction division of more than one fraction
(9/2) / (28 /9) where "/" represents the division sign
Appreciating the order of precedence is crucial when evaluating expressions involving fractions. The order of precedence dictates the sequence in which operations should be carried out. In the case of fractions, calculations within parentheses should be performed first, followed by multiplications and divisions from left to right, and finally additions and subtractions from left to right. Adhering to the correct order of precedence ensures accurate and consistent results.
Thoroughly understanding these concepts and mastering the techniques to add, subtract, multiply, and divide fractions allows individuals to confidently solve various mathematical problems and build a solid mathematical foundation.
Enter the expression below to solve complex mixed number fraction
[ 7(1/8) ] - [ 5(3/7) ]
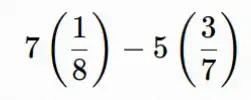
Enter the expression below to solve complex mixed number fraction of fraction multiplication
[ 1(3/5) ] x [ 2(1/3) ] x [ 3(3/7) ]
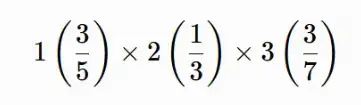
Choose the appropriate problem from the prompt drop-down
Input your equation into the designated text box (plz! refer to the expression to correctly enter the expression)
Click on the solve button
Your worksheet will be generated accordingly
Click on the worksheet to copy your answer to clipboard
Changes improper to mixed number fractions
The solver ensures accurate results every time.
It can calculate the addition, subtraction, multiplication and division fractions
The solver is useful for both students and professionals to solve complex order of precedence fractional problems
